Table of Contents |
The goal of this challenge will be to write exponential functions in the form 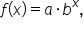 where
where 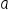 is a nonzero number and b is a positive number not equal to 1.
is a nonzero number and b is a positive number not equal to 1.
Earlier, you may recall, we saw situations in which a constant percent increase or decrease can be modeled by an exponential function.
EXAMPLE
Suppose the value of a house is predicted to increase by 3.4% per year for the foreseeable future. If its value now is $280,000, then what is an exponential model for the value of the home x years from now?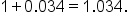
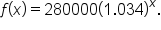
Here is another example where a quantity is decreasing over time.
EXAMPLE
After taking 100 milligrams of medicine, the quantity of medicine in one’s bloodstream decreases by 30% every hour. What is the exponential function that models the amount of medicine remaining in the bloodstream after t hours?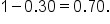
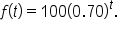
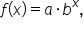 considering for now
considering for now 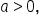 we observe these behaviors:
we observe these behaviors:
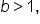 the value of
the value of 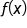 increases as x increases.
increases as x increases. 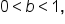 the value of
the value of 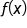 decreases as x increases.
decreases as x increases. 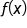 when
when 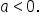
When the growth (or decay) rate is unknown, we need to find 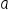 and b in the equation
and b in the equation 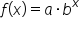 some other way. Since there are two values to find, we can use two solution points to write the equation.
some other way. Since there are two values to find, we can use two solution points to write the equation.
EXAMPLE
Write the equation of an exponential function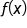 that contains solution points
that contains solution points 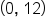 and
and 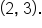
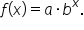
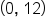 is a solution point, this means
is a solution point, this means 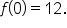
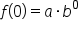
|
Replace x with 0. |
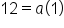
|
Replace 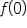 with 12, and simplify with 12, and simplify 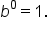
|
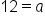
|
Solve for 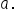
|
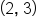 is a solution point, this means
is a solution point, this means 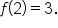
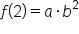
|
Replace x with 2. |
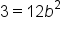
|
Replace 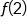 with 3 and with 3 and 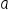 with 12. with 12.
|
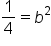
|
Divide both sides by 12 and simplify. |
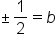
|
Apply the square root principle. |
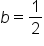
|
The value of b must be positive according to the definition of an exponential function. Therefore, 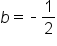 is not considered. is not considered.
|
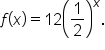
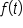 has solution points
has solution points 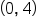 and
and 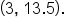
Notice how the previous problems were very nice to solve since the point 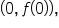 the y-intercept, was known. When the y-intercept is not known, more advanced algebraic techniques are required.
the y-intercept, was known. When the y-intercept is not known, more advanced algebraic techniques are required.
EXAMPLE
Determine the equation of an exponential function that contains solution points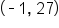 and
and 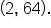
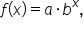 substitute each solution pair in order to find
substitute each solution pair in order to find 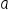 and b.
and b.
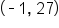 is a solution point, it follows that
is a solution point, it follows that 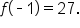
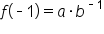
|
Replace x with -1. |
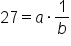
|
Replace 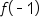 with 27, and rewrite with 27, and rewrite 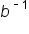 as as 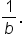
|
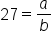
|
Rewrite the right-hand side as one fraction. |
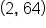 is a solution point, it follows that
is a solution point, it follows that 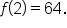
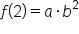
|
Replace x with 2. |
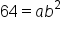
|
Replace 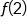 with 64. with 64.
|
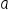 and b. These are not linear equations as we are accustomed to, but we can still solve using the substitution method.
and b. These are not linear equations as we are accustomed to, but we can still solve using the substitution method.
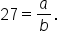 Multiplying both sides by b gives
Multiplying both sides by b gives 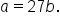 This can now be substituted into the other equation, which can then be solved for b.
This can now be substituted into the other equation, which can then be solved for b.
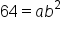
|
Start with this equation. |
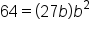
|
Substitute 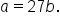
|
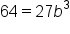
|
Simplify the right-hand side. |
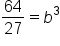
|
Divide both sides by 27. |
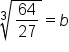
|
Take the cube root of both sides. |
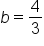
|
Simplify. |
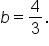 Substituting into the equation
Substituting into the equation 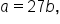 we find
we find 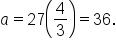
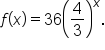
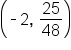 and
and 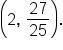
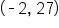 and
and 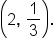
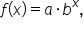 where
where 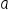 is a nonzero number and b is a positive number not equal to 1, using two different methods: If known, you can use the starting value and corresponding percent increase and decrease, or you can write the equation given two solution points. It is important to note that when solving for the base b, remember that b has to be positive.
is a nonzero number and b is a positive number not equal to 1, using two different methods: If known, you can use the starting value and corresponding percent increase and decrease, or you can write the equation given two solution points. It is important to note that when solving for the base b, remember that b has to be positive.
SOURCE: THIS WORK IS ADAPTED FROM PRECALCULUS BY JAY ABRAMSON. ACCESS FOR FREE AT OPENSTAX.ORG/BOOKS/PRECALCULUS/PAGES/1-INTRODUCTION-TO-FUNCTIONS