Use Sophia to knock out your gen-ed requirements quickly and affordably. Learn more
Become a Member Try for Free
×

The Vertex Formula

Author: Sophia
PDF Version

what's covered
This tutorial covers how to find the vertex of a parabola, using the vertex formula, through the definition and discussion of:

Table of Contents

1. Vertex

A parabola is the term used for a graph of a quadratic equation, which can generally be written as:

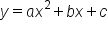
y equals a x squared plus b x plus c

If the coefficient a is positive, the graph is a U shape pointing up. If the coefficient a is negative, the graph is a U shape pointing down. A parabola will have a maximum point if the graph is pointing down or a minimum point if the graph is pointing up. The maximum or minimum point of a parabola is called the vertex.

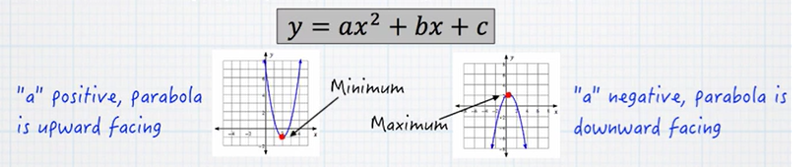
The vertex provides useful information about the highest or lowest value in a quadratic relationship. These highest or lowest values are related to optimization problems, such as maximum profit or minimum cost.

2. The Vertex Formula

You can use the equation for a parabola to find the vertex without looking at the graph. When doing so, it’s important to remember the following points about a parabola:

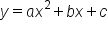  • A parabola is the graph of a quadratic equation in the form: y equals a x squared plus b x plus c.
  • When the coefficient a is positive, the parabola will be upward-facing. When the coefficient a is negative, the parabola will be downward-facing.
  • The vertex is the maximum or minimum point on the parabola.
The x-coordinate of the point of the vertex can be found using the following formula, in which a and b are the coefficients in your quadratic equation.

formula to know
x-coordinate of Vertex
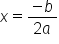
x equals fraction numerator negative b over denominator 2 a end fraction

Once you have used the formula to find the x-coordinate of the vertex, you can substitute this value in for x in the equation to determine the y-coordinate of the vertex.

3. Finding the Vertex

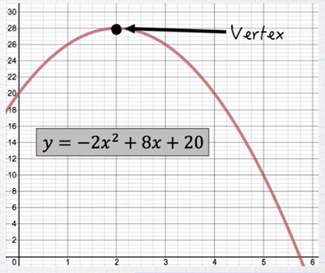
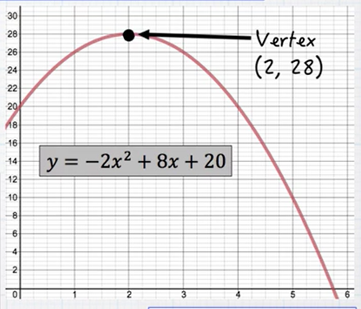
Suppose an Olympic diver is competing for a medal. Her dive can be modeled by the equation and graph shown below, where x is the time in seconds after she begins the dive and y is the height in feet above the water. In this example, the vertex of the parabola is a maximum point, so by finding the vertex using the vertex formula, you can determine the maximum height of her dive.

First, you take your values for a and b from your equation, and substitute them into the formula for the x coordinate of the vertex (the vertex formula).

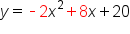
y equals short dash 2 x squared plus 8 x plus 20

In this equation, we have:

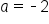
  • a equals short dash 2
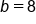
  • b equals 8
Using the formula, this gives us:

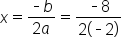
x equals fraction numerator short dash b over denominator 2 a end fraction equals fraction numerator short dash 8 over denominator 2 open parentheses short dash 2 close parentheses end fraction

Multiply and divide the values accordingly to provide x equals 2, so the x-coordinate of your vertex is 2.

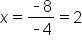
x equals fraction numerator short dash 8 over denominator short dash 4 end fraction equals 2

Next, substitute 2 into your original equation for x to find the y-value of the vertex.

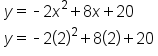
table attributes columnalign left end attributes row cell y equals short dash 2 x squared plus 8 x plus 20 end cell row cell y equals short dash 2 left parenthesis 2 right parenthesis squared plus 8 left parenthesis 2 right parenthesis plus 20 end cell end table

Simplify with your exponent, then multiply and add, according to the order of operations.

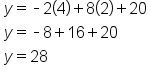
table attributes columnalign left end attributes row cell y equals short dash 2 left parenthesis 4 right parenthesis plus 8 left parenthesis 2 right parenthesis plus 20 end cell row cell y equals short dash 8 plus 16 plus 20 end cell row cell y equals 28 end cell end table

The y-coordinate of your vertex is 28, and you can see that your vertex is at the point (2, 28). This means that 2 seconds after she begins the dive, she reaches her maximum height of 28 feet above the water.

IN CONTEXT

Natasha kicks a soccer ball during a game. How can you determine the maximum height of the ball?

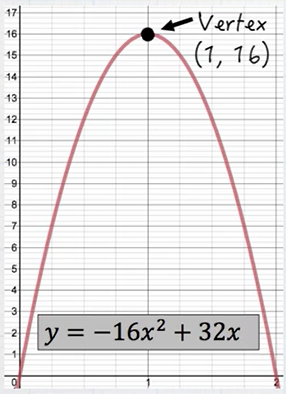
Well, the flight of the ball can be modeled by the equation and graph shown below, where x is the time in seconds after she kicks the ball and y is the height of the ball in feet. Note that the vertex is a maximum point.



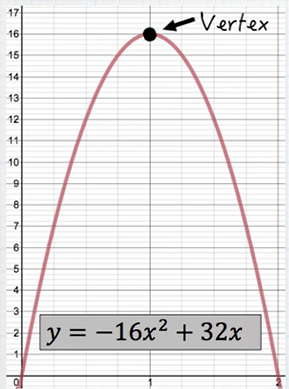
You can start by using your formula for the x-coordinate of the vertex and substituting your values in for b and a.

y equals short dash 16 x squared plus 32 x

In this equation, we have:
  • a equals short dash 16
  • b equals 32
Using the formula, this gives us:

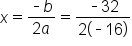
x equals fraction numerator short dash b over denominator 2 a end fraction equals fraction numerator short dash 32 over denominator 2 open parentheses short dash 16 close parentheses end fraction

Simplify the denominator and divide these values to provide the value of the x-coordinate of the vertex, which is 1.

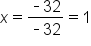
x equals fraction numerator short dash 32 over denominator short dash 32 end fraction equals 1

Next, substitute 1 in for x in your original equation to find the y-coordinate of the vertex.

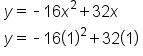
table attributes columnalign left end attributes row cell y equals short dash 16 x squared plus 32 x end cell row cell y equals short dash 16 left parenthesis 1 right parenthesis squared plus 32 left parenthesis 1 right parenthesis end cell end table

Simplify your exponent, multiply, and add.

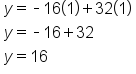
table attributes columnalign left end attributes row cell y equals short dash 16 left parenthesis 1 right parenthesis plus 32 left parenthesis 1 right parenthesis end cell row cell y equals short dash 16 plus 32 end cell row cell y equals 16 end cell end table

The y-coordinate of your vertex is 16. Therefore, your vertex is at the point (1, 16). The maximum height of the ball is reached after one second and the maximum height is 16 feet.

summary
Today you learned that the maximum or minimum point of a parabola is called the vertex, noting that a parabola, the graph of a quadratic equation, will have a maximum point if the graph is pointing down or a minimum point if the graph is pointing up. You also learned that you can find the vertex of a parabola using the vertex formula, by determining the x-coordinate of the point of the vertex. Once you have the x-coordinate of the vertex, you can then substitute this value in for x in the equation to find the y-coordinate of the vertex.

Source: THIS TUTORIAL WAS AUTHORED BY Colleen Atakpu FOR SOPHIA LEARNING. PLEASE SEE OUR TERMS OF USE.

Formulas to Know
x-coordinate of Vertex

x equals fraction numerator short dash b over denominator 2 a end fraction

© 2025 SOPHIA Learning, LLC. SOPHIA is a registered trademark of SOPHIA Learning, LLC.