Table of Contents |
Exponential equations can be equivalently written using a logarithm. In general, we can say that the follow two equations are equivalent:
| Exponential Equation | Logarithmic Equation |
|---|---|
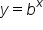
|
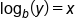
|
Notice that the base of the exponential expression becomes the base of the logarithmic expression. Also notice that y, which was the output of the exponential equation, is the input of the logarithmic operation. This is characteristic of inverse relationships.
When solving exponential equations using logarithms, we often apply two important properties of logarithms: the power property of logs and the change of base property of logs:
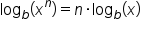
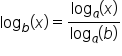
We most often use the change of base property when we wish to use our calculators to evaluate logs. This is because most calculators can only evaluate logs in base 10 (the common log) or e (the natural log).
EXAMPLE
Solve the exponential equation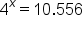 .
.
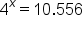
|
Rewrite using a logarithmic eqution |
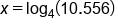
|
Equivalent equation to 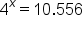
|
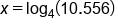
|
Apply the Change of Base Property of Logs |
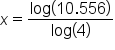
|
Use calculator to evaluate |
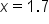
|
Our solution |
As with all equations, we can apply inverse operations to both sides of the equation in order to isolate the variable. The inverse operation of an exponent is the logarithm. In this next example, we will see how we can apply the logarithm and other inverse operations to isolate the variable.
EXAMPLE
Solve the exponential equation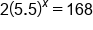 .
.
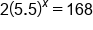
|
Divide by 2 to have only 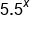 on the left side on the left side
|
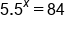
|
Apply log of both sides |
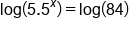
|
Apply the Power Property of Logs |
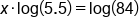
|
Divide both sides by 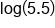
|

|
Use calculator to evaluate |
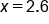
|
Our solution |
Source: THIS TUTORIAL HAS BEEN ADAPTED FROM "BEGINNING AND INTERMEDIATE ALGEBRA" BY TYLER WALLACE. ACCESS FOR FREE AT www.wallace.ccfaculty.org/book/book.html. License: Creative Commons Attribution 3.0 Unported License