Table of Contents |
Working with the quadratic formula is one method in determining if there are no real solutions to a quadratic equation. Recall the quadratic formula:
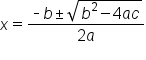
The expression that is underneath the square root is called the discriminant. Because the discriminant is underneath a square root sign, it must not have a negative value, otherwise the square root does not evaluate to a real number. This is how we can tell if a quadratic has no real solutions by using the quadratic formula.
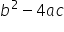
The following table shows the different types of solutions when the discriminant is positive, zero, and negative.
Discriminant (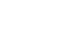 ) )
|
Type of Solutions |
|---|---|
| Positive | Two Real Solutions |
| Zero | One Real Solution |
| Negative | Two Complex Solutions |
In addition, if the discriminant is a perfect square (such as 16 or 81), the quadratic equation has two rational solutions (the solutions will not contain radicals).
EXAMPLE
Find the discriminant for the quadratic equation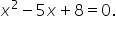 Then determine if the equation has real or nonreal solutions.
Then determine if the equation has real or nonreal solutions.
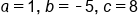
|
Identify the values to substitute into the discriminant formula. |
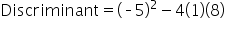
|
Substitute the values of 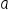 , b, and c. , b, and c.
|

|
Simplify the expression. |
Even though some quadratic equations may have no real solutions, we can still express their solutions mathematically. To do so, we use the imaginary number, i, in the expression for its solution. The imaginary number, i, is a non-real number that represents the square root of -1.
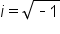
The letter i is used to denote the square root of negative 1. We can rewrite the square roots of negative numbers using this letter.
EXAMPLE
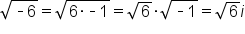
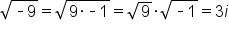
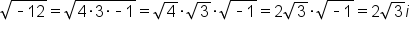
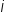 ).
).If we encounter a negative value underneath the radical when using the quadratic formula, we can express the solutions to the quadratic equation using complex numbers. A complex number contains a real part and an imaginary part, such as 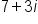 or
or 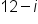 .
.
EXAMPLE
Suppose we were calculating the solutions to a quadratic equation and got to this step: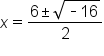
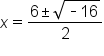
|
Rewrite square root |
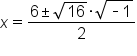
|
Evaluate the square root of 16 and -1 |
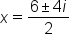
|
Create two separate solutions, one addition and one subtraction |
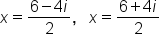
|
Divide each term by 2 |
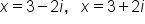
|
Our solutions |
Source: THIS TUTORIAL HAS BEEN ADAPTED FROM "BEGINNING AND INTERMEDIATE ALGEBRA" BY TYLER WALLACE. ACCESS FOR FREE AT www.wallace.ccfaculty.org/book/book.html. License: Creative Commons Attribution 3.0 Unported License