Table of Contents |
When we graph a function, we are considering the entire function. What if we only wanted part of the graph?
EXAMPLE
Consider the function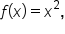 and several “pieces” of the graph, as shown below:
and several “pieces” of the graph, as shown below:
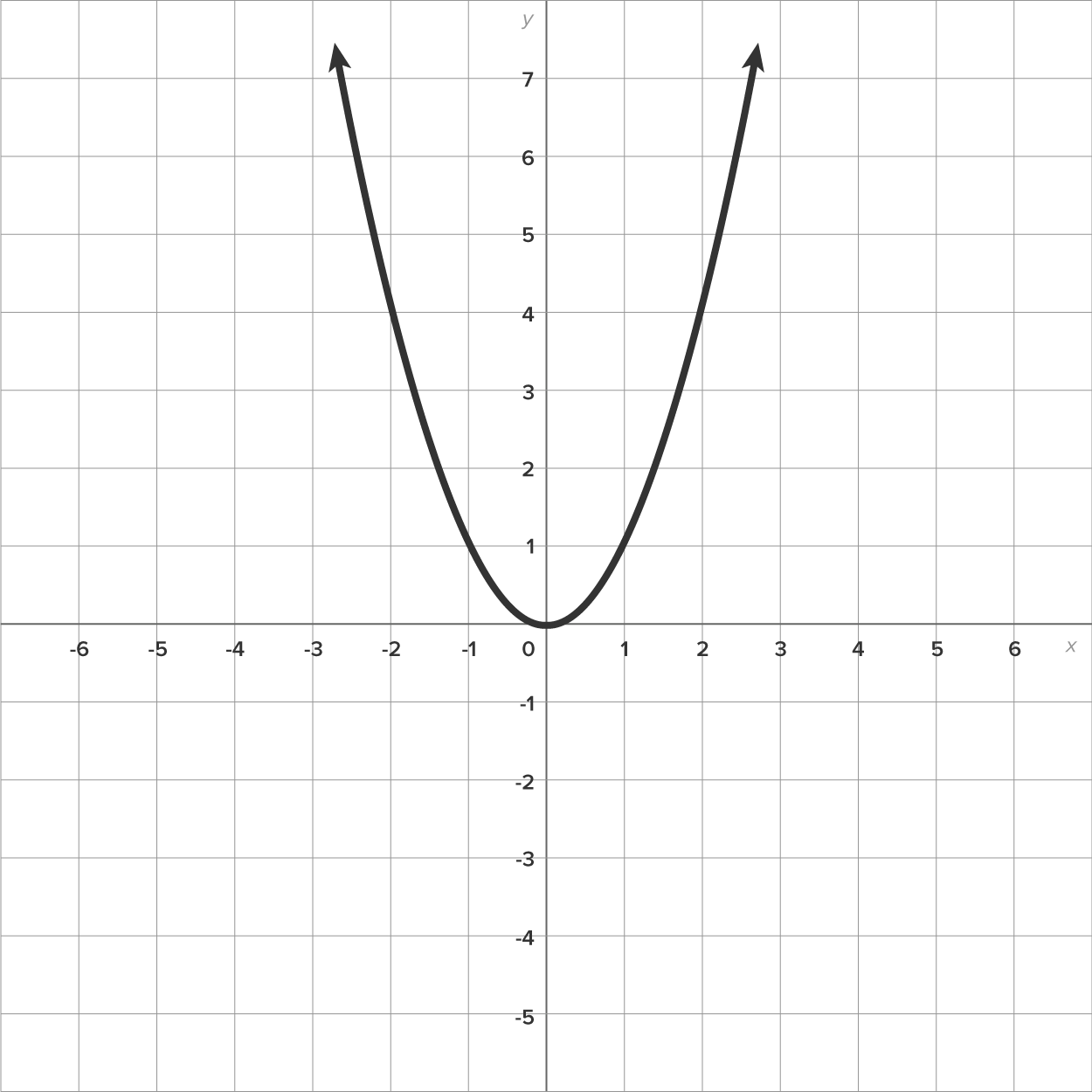
Graph 1:  (entire graph) (entire graph)
|
Graph 2: 
|
|---|---|
|
|
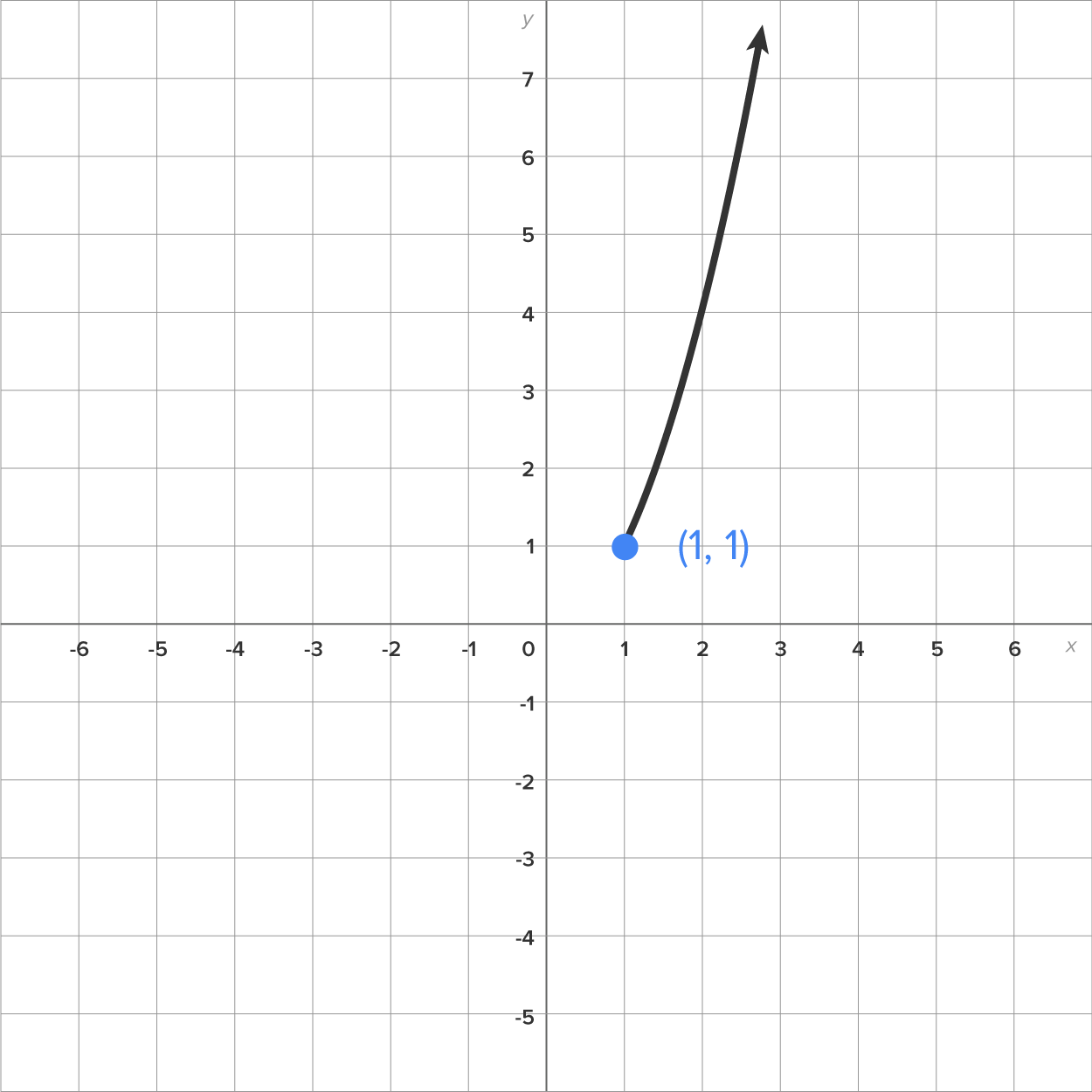
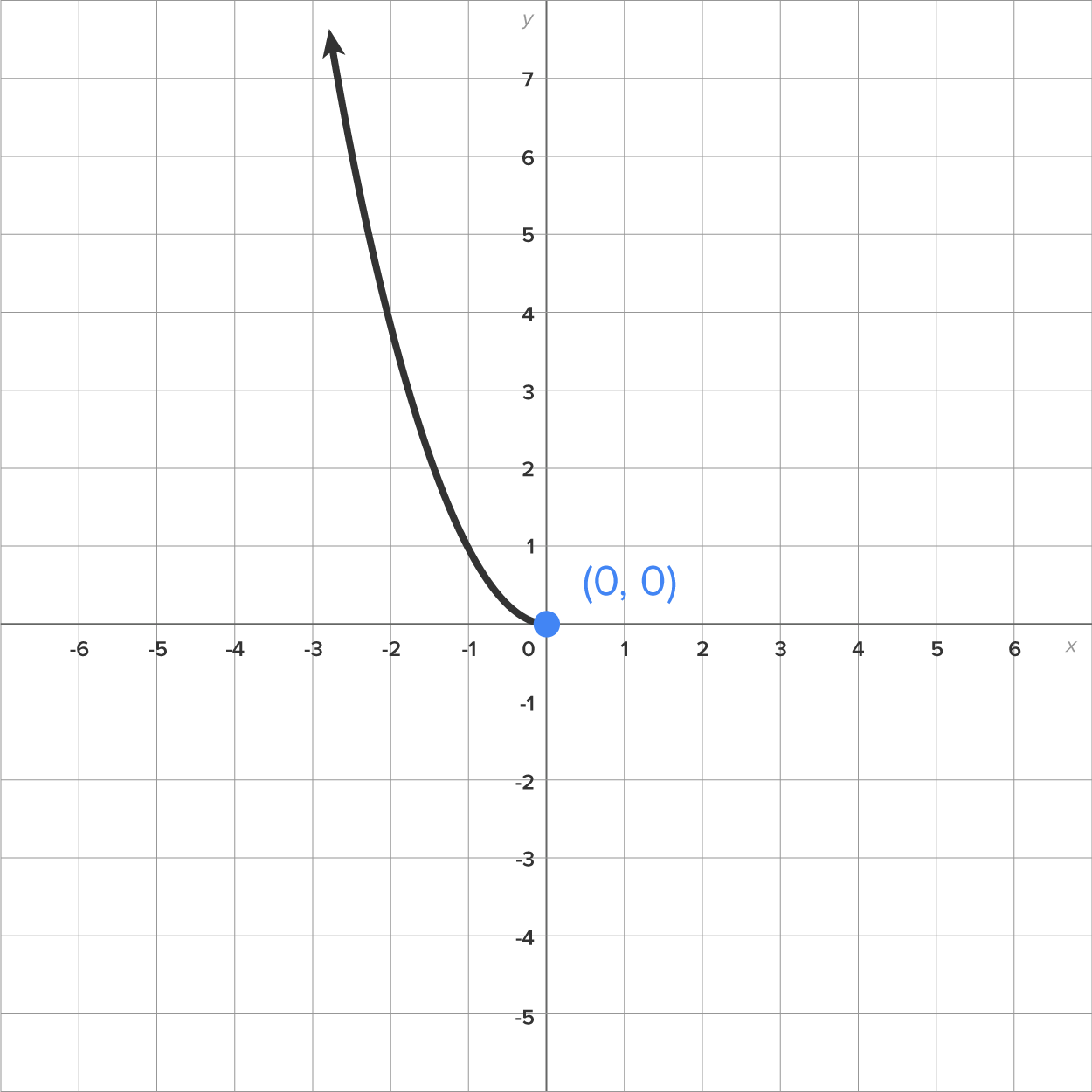
Graph 3: 
|
Graph 4: 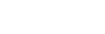
|
|
|
For instance, in Graph 3 above, the 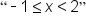 is the domain restriction since it is not the entire domain of
is the domain restriction since it is not the entire domain of 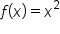 (which is all real numbers).
(which is all real numbers).
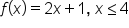
A piecewise function is made up of other functions that are on restricted domains. For instance, consider the function:
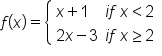
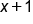 ”, but only if the input is less than 2, and to use “
”, but only if the input is less than 2, and to use “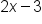 ” if the input is at least 2.
” if the input is at least 2.
This means that the graph of the function will be “part of” the graph of 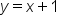 along with “part of” the graph of
along with “part of” the graph of 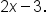 Here is how we put this together:
Here is how we put this together:
|
|
|---|---|
|

|
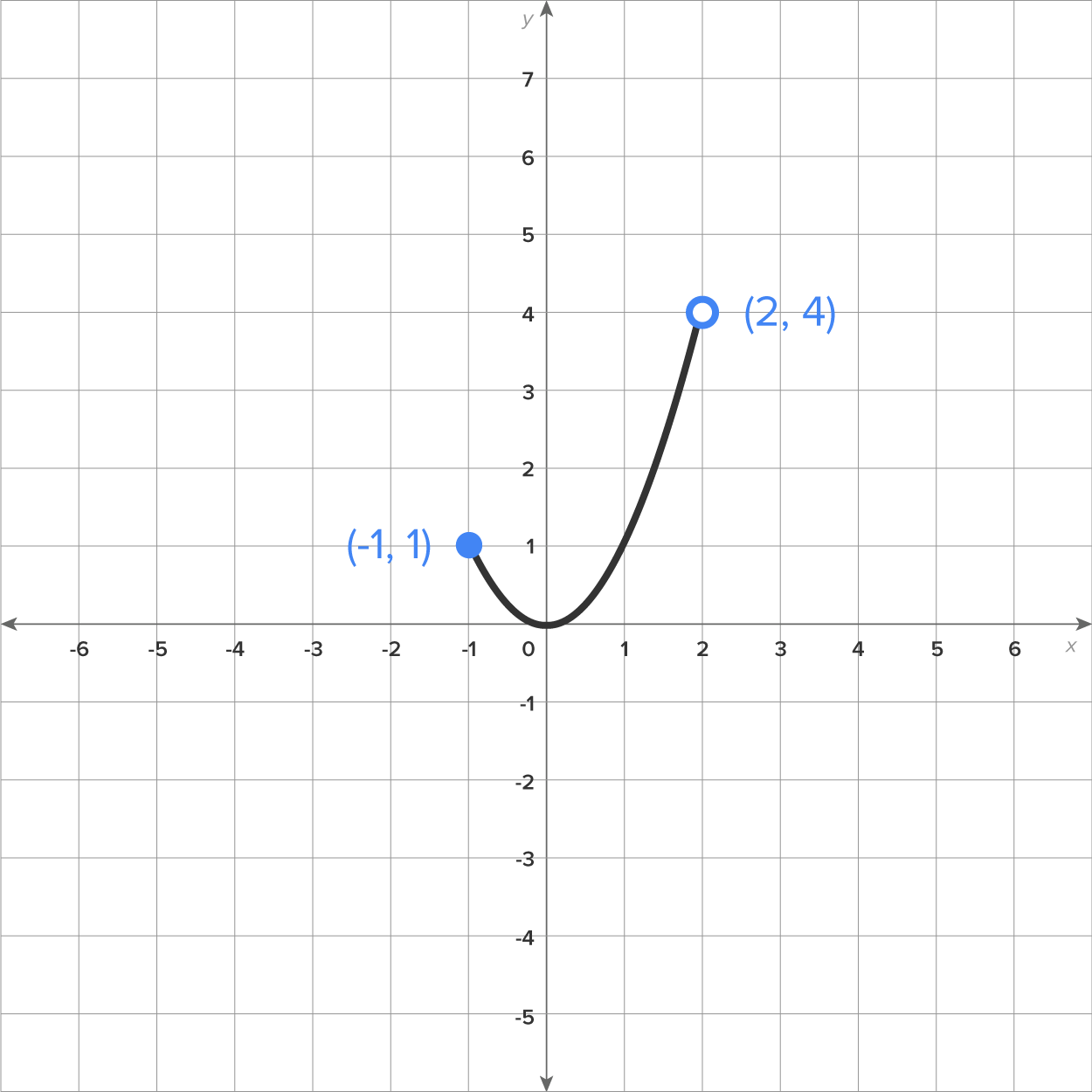
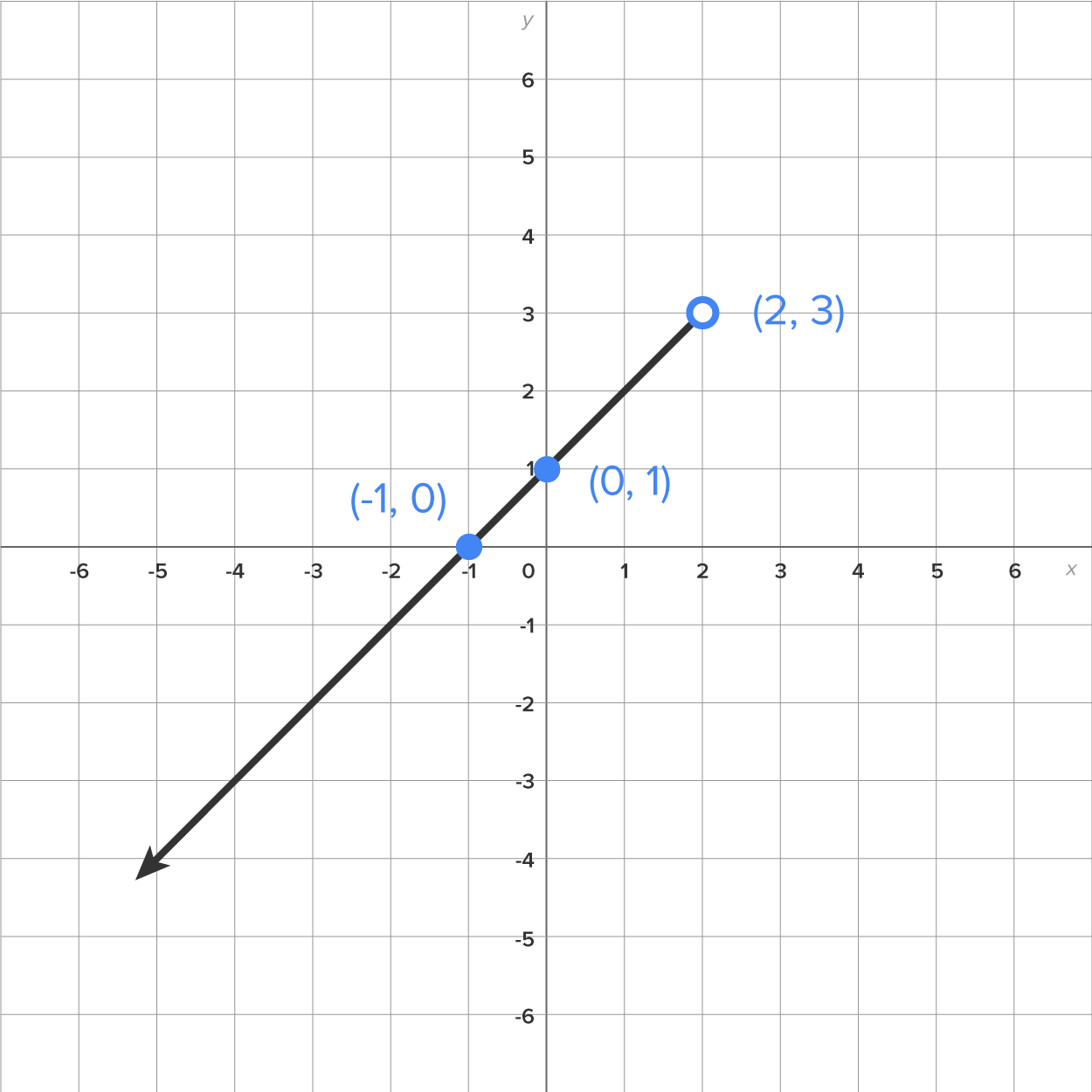
The graph of  is these pieces put together on one graph as follows:
is these pieces put together on one graph as follows:
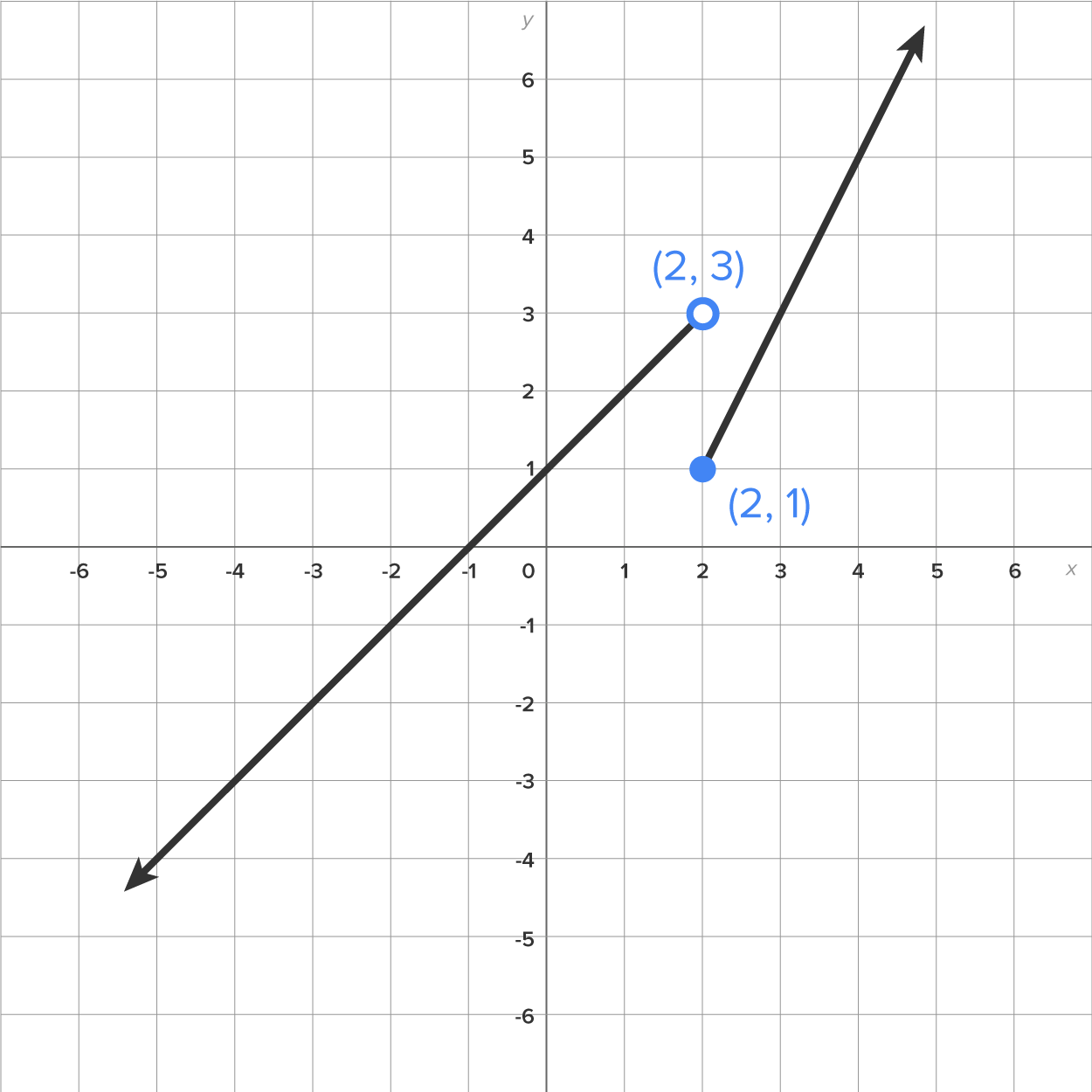
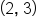 and a closed circle at
and a closed circle at 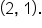 If both circles were closed, then the graph would not represent a function. A common error is to close both circles at the breaking point, so be sure to double check which one is included and which one is not, since both cannot be included!
If both circles were closed, then the graph would not represent a function. A common error is to close both circles at the breaking point, so be sure to double check which one is included and which one is not, since both cannot be included!
SOURCE: THIS TUTORIAL HAS BEEN ADAPTED FROM 1) CHAPTER 0 OF "CONTEMPORARY CALCULUS" BY DALE HOFFMAN. ACCESS FOR FREE AT WWW.CONTEMPORARYCALCULUS.COM. LICENSE: CREATIVE COMMONS ATTRIBUTION 3.0 UNITED STATES. 2) OPENSTAX "PRECALCULUS” BY JAY ABRAMSON. ACCESS FOR FREE AT OPENSTAX.ORG/DETAILS/BOOKS/PRECALCULUS-2E. LICENSE: CREATIVE COMMONS ATTRIBUTION 4.0 INTERNATIONAL.