Finding the Sum of an Arithmetic Sequence

1. Formula for the Sum of an Arithmetic Sequence

Consider the arithmetic sequence {3, 8, 13, 18, 23, 28}.

With only a few terms, it is easy enough to find the sum of all terms in this sequence. We can simply add the terms together to get a sum of 93. However, what if we had been given a sequence with 100 terms in it, and asked to find the sum? We still could add the terms concretely, but it would be inefficient. Let's take a look at an interesting shortcut.

	Add the outermost terms: 3+28=31
	Working our way in: 8+23=31
	Further in: 13+18=31

There is an interesting pattern when we add the first and last terms together, and then work our way to the center of the sequence. In each case, the sum is the same, in this case, 31. Due to this pattern, we know that the sum of all of the terms is going to be a multiple of 31. How many times should we multiply 31? We saw that we formed 3 pairs of 31, so the sum of all 6 terms is 93. To generalize this pattern, we need to think about the relationship between 6 terms and 3 pairs that sum to the same value. Since we were pairing terms, there are exactly half as many pairs as there are terms.

In general, then, to find the sum of an arithmetic sequence, we can add the first term and the n-th term, and then multiply that by the number of terms, n, divided by 2. Here is what our formula looks like:

formula to know

Sum of an Arithmetic Sequence

In this formula, we can define each variable as:

• : the sum of n terms
• : the value of the 1st term
• : the value of the nth term
• : the term

2. Finding the Sum when a_n is Given

EXAMPLE

Find the sum of the first 8 terms in the sequence:

Because we are summing the first through eighth terms of the sequence, we need to identify the first term () and the eighth term () to plug into our formula, which in this case are and

We also need to know the value of n for our formula. Again, since we are summing eight terms, so

Now we can make all appropriate substitutions into our sum formula and solve:

	Substitute , ,
	Add -8 and 34
	Divide 8 by 2
	Multiply 4 and 26
	Our Solution

3. Finding the Sum when a_n is Not Given

In our final example, we are going to find the sum of a sequence when we aren't initially given the value of the nth term (or the last term in the sequence we are summing). The only difference between this example and the previous example is that we will need to calculate the value of the nth term ourselves. This isn't too difficult, because we have already learned the formula for that as well.

formula to know

Arithmetic Sequence

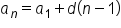
Recall that:

• : the value of the nth term
• : the value of the 1st term
• : the common difference
• : the term

EXAMPLE

Find the sum of the first 100 terms of the following sequence:

In order to make use of our formula for the sum, we first need to know the value of the first term and the value of the 100th term. We know the first term is 4, but we need to find the value of the 100th term. Let's use our formula to find the value of any term. This formula relies on knowing the common difference, or constant value that is added to get from one term to the next. We can quickly find the common difference by finding the difference between any two consecutive terms. Let's choose 18 and 25. The difference between these two numbers is 7. So 7 is d, the common difference in our first formula.

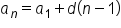
	Substitute , ,
	Subtract 1 from 100
	Multiply 7 and 99
	Add 4 and 693
	This is the value of the 100th term

We aren't done yet. We just found the value of the 100th term that we need to be able to use the formula for finding the sum of the first 100 terms to the sequence. Now that we have all of the information we need, we can apply the sum formula:

	Substitute , ,
	Add 4 and 697
	Divide 100 by 2
	Multiply 50 and 701
	Our solution

This tells us that the sum of the first 100 terms in the arithmetic sequence is 35,050.

summary

When you want to find the sum of a sequence, it can be inefficient to actually sum the values of each term. Instead, we can use a formula for the sum of an arithmetic sequence. In this formula, n is the number of terms that you're summing, is the first term, and is the last or the nth term that you want to sum. When finding the sum when a_n is given, we just plug in the first and nth term into the formula. When finding the sum when a_n is not given, we don't have the nth term. We can determine by using the formula for an arithmetic sequence, where is the first term, d is the common difference, and n is the number of terms that you are summing.