Combining Like Terms

1. Terms and Like Terms

Solving an equation for a single variable is not always a single-step process. Often, complex expressions appear on either side of an equation, and it requires several steps to isolate the variable. It can save time and prevent confusion if you can simplify the expression by combining like terms before attempting to isolate the variable.

A term is a collection of numbers, variables, and powers combined through multiplication. Terms are referred to by their variable and power or exponent.

Consider the following expression with three terms:

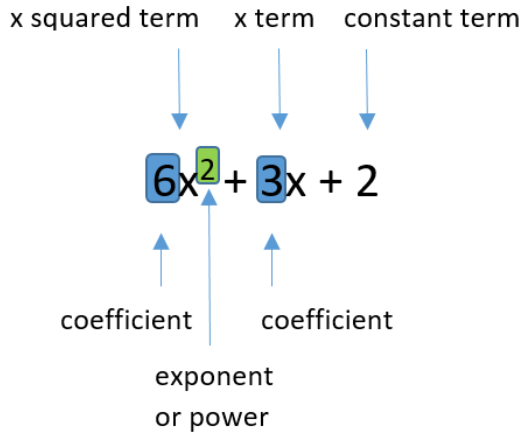
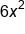• In this expression, the first term, , can be read as 6 x squared, and is called an x-squared term. The 6 in front of the x squared is called a coefficient. The 2 is the exponent or power of the term.
• The second term, 3x, is called an x term. The coefficient of this term is 3. Here the variable x has no written exponent, so the implied exponent is 1 ().
• The last term, 2, is a constant term. Terms without a variable are called constant terms because the value of that term will be the same no matter what the variable is.

Like terms have the same variables, with each variable having the same exponent. In the expression above, there are no like terms, because in each term, the variable x has a different exponent. However, in the following expression, 3x and 5x are like terms, and you can simplify the expression by combining these two terms.

terms to know

Term

A collection of numbers, variables, and powers combined through multiplication

Like Terms

Terms involving the same variables with each variable having the same exponent

---

2. Combining Like Terms

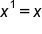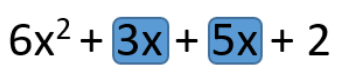
Combining like terms is a method to simplify expressions before solving the equation using inverse operations. Terms may only be added or subtracted if they are like terms. Like terms are combined by adding or subtracting their coefficients and leaving the variable and exponent the same. A coefficient is the number that appears in front of a variable and acts as a factor or multiplier. Coefficients can be any real number, and terms without a written coefficient have an implied coefficient of 1, as illustrated here:

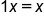
There are several common mistakes to avoid when combining like terms:

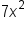• The exponent does not change when combining like terms. For example, 4x plus 3x does not equal , because the exponent does not change. Instead, 4x plus 3x means that you are adding 4 xs and 3 more xs, which equals a total of 7 xs. Thus, 4x plus 3x equals 7x.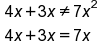
  
  
  
  
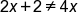
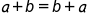
• You cannot combine terms that are not like terms. For example, 2x plus 2 does not equal 4x. Even though both terms have a 2, they are not like terms, because their variable and variable exponent are not the same. Therefore, 2x and 2 cannot be combined or simplified.
  
  
  

When combining like terms, it’s useful to remember the commutative property for addition, which states that a plus b is equal to or the same as b plus a. In other words, you can add real numbers in any order. This property is useful when simplifying expressions involving like terms, because it allows the terms to be rearranged when you combine like terms using addition and subtraction.

formula to know

Commutative Property of Addition

EXAMPLE

Suppose you are simplifying the expression: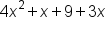

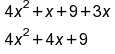
In it, you have two like terms, x and 3x. The term x has an implied coefficient of 1, even though it is not written. To combine the terms, you add the coefficients 1 and 3. 1x plus 3x is 4x, so your expression becomes:

How would you combine like terms in a more complex expression with varying terms?

EXAMPLE

Combine like terms in the expression: .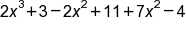

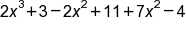
	Our Expression
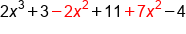
	First, there are two x squared terms. The subtraction sign in front of the 2x squared makes the term negative. Combine -2x with 7x squared to get 5x squared.
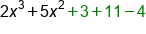
	Next, we have three constant terms, 3, 11 and negative 4. Combine these terms to get 10.
	Our Simplified Expression

A complex expression involving both varying terms and fractions requires several steps in the simplification process.

EXAMPLE

Suppose you want to simplify the expression: .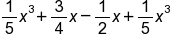

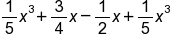
	Our Expression
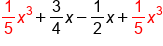
	There are two x cubed terms. These can be combined. Since the denominators are the same, we can add the numerators and keep the denominators. 1/5 and 1/5 equals 2/5x cubed.
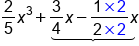
	There are two x terms. Since the denominators are not the same, we need to find a common denominator. The least common denominator would be 4, so we can leave the fraction 4/3 unchanged, and multiply the fraction 1/2 by 2 in both the numerator and the denominator.
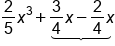
	Now that we have the same denominator, we can subtract 3/4x and 2/4x, which is 1/4x
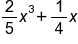
	Our Simplified Expression

term to know

Coefficient

The number in front of a variable term that acts as a factor, or multiplier